Authors: brilliant predecessors in my interpretation.
Composed by VVvv. Translated by Google translate, ran by VVvv.
Thanks are given to professors Jiri Bicak and Michal Krizek.
Tenerife – Miraverde version, June 10, 2021
Translated August 18, 2021
In Looking into Universe, the consequences of using a curved space model with constant curvature to model the space of the Universe as a whole are elaborated in four parts, in four stages. In the first part, the consequences for light propagation and observation in such a space are explained. In the second part, the consequences of the expansion of such a space are described. In the third part, the consequences of a broader understanding of the space-time modeled in this way are presented, and in the fourth part, a comparison of the consequences of the closed and open space model is elaborated.
In the first part Looking into Universe.1 {2NdV.1_EN}, we start from the comparison of a circle, as a representative of a constantly curved 2D space, which appears to us as a one-dimensional space from the inside, with the surface of a sphere as a representative of a 3D space, which appears to us as a two-dimensional space from the inside and finally from some 4D constantly curved shape called KOZAK, whose surface appears to us as a three-dimensional space from the inside.
In order to observe in such a space, we advantageously use a description using a spherical coordinate system (r, φ, ψ), since from any point in such a space we could observe from the center of such a coordinate system in directions that are any combination of direction angles φ and ψ. Thus, observations in the directions left/right and up/down, and movement would add a third direction, forward/backward, i.e. along the radial coordinate r along which the light propagates to us.
Since a straight movement in any closed space would get us back to the starting point, but from the opposite direction, we called such a movement in a constantly curved space as a movement along a SUBSTITUTIVE CIRCLE, which substitutes such a straight direction. Looking from the outside, the strait direction in such a space, and therefore the propagation of light, is considered to follow such a circle.
The propagation of light to the observer in a constantly curved space is schematically captured in the sketch called SPACE 4D in Cross section [2P4DvP_EN]:
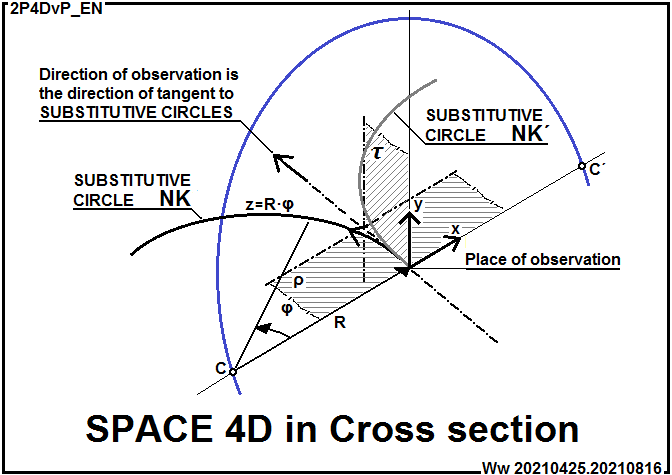
For one place of our observation in one chosen direction, here in the direction of coordinate z, the straight direction lies in the section plane ρ (horizontally hatched in the figure) represented by thearcs of two SUBSTITUTIVE CIRCLES NK and NK´ of radius R.
Then our observation forward along the arc of such circles has the coordinate z=R•φ, where the angle φ is measured in the arc measures with the origin at the point of observation. If we were to move along such an arc for long enough, then for the angle φ=2π the arc would close into a complete circle, and we would get back to our starting point but from the opposite side.
It is already clear from the picture that the light that would propagate to us along the marked arc, our eye can no longer distinguish from the light that would propagate to us along the marked tangent to the arc. And that is exactly why we can never directly observe the curvature of spaces from the inside.
But let´s be aware that in a given direction of observation we can lead a huge number of such SUBSTITUTIVE CIRCLES, which we would obtain by gently rotating the plane ρ of both drawn SUBSTITUTIVE CIRCLES around the direction of observation. The centers C of such SUBSTITUTIVE CIRCLES would describe the arc marked in blue.
For an ideal space of constant curvature, the probability of our view and/or movement would be absolutely equal for all SUBSTITUTIVE CIRCLES formed in our direction of observation. But in real space, our actual observation and/or actual movement forward would be reduced to one single circle. The sum of the probabilities of the occurrence of individual circles, which would mean certainty, would “collapse” (as this phenomenon is called in physics) into one probability, i.e. into the certainty that would affect us.
Let´s imagine the same thing in a space one unit smaller. It is as if we were standing on the surface of the (Earth-)sphere and thinking about which direction we should go on our journey, when all directions would be equally valued for us. And only after taking a step would all the same probabilities of directions “collapse” into the probability of one, into the certainty of the direction in which we set out on our journey.
The consequences for our observations in a constantly curved space are captured in the figure STARS and LIGHT PROPAGATION G [2phG_EN], where the situation just described in our section ρ is drawn as sketch 1:
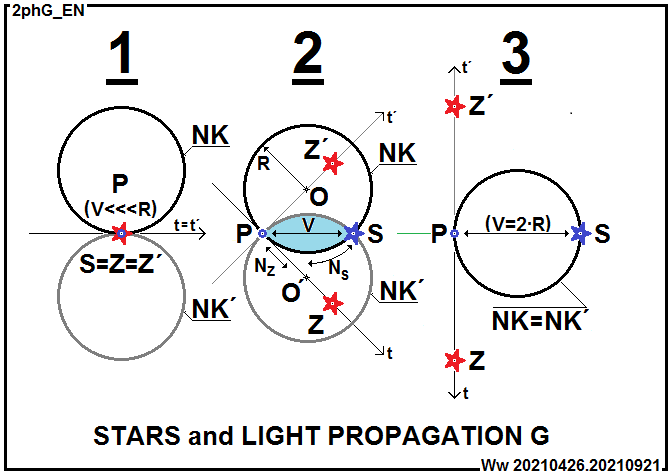
The direction of observation is drawn from left to right. The observed object, here as a star in its actual position “S“, is observed by us from the place “P” at such a small distance “V” (V<<<R) that the influence of the curvature of space would be practically negligible, so it would be observed at common tangent “t=t´” to SUBSTITUTIVE CIRCLES “NK” and “NK´“.
For larger distances “V“, the situation is drawn on sketch 2, for which the situation is chosen here, where the tangents “t” and “t´” to the SUBSTITUTIVE CIRCLES “NK” and “NK´” form a right angle of 90° together. But the observer from the point “P” observes the star along the tangents in the apparent positions “Z” and “Z´“.
Since we chose the section ρ arbitrarily, by rotating the section around the PS axis, we would observe more and more pairs of apparent positions of the star, and after fine enough rotation, we would observe an illuminated circle formed by the rotating the “Z” and “Z´” positions around the P-S axis. The common enclosed space created by the rotation of the circles would be a rotationally symmetrical shape resembling some pointed rugby ball of constant curvature. That is, a kind of light blue marked rugby ball, which we will therefore call abbreviated as “rugball” for simplicity.
In real space, which cannot be absolutely constantly curved, because unevenly distributed gravity has deformed it into a space with a locally fluctuating amount of curvature, the illuminated circle would break up into discrete locations from which the light would fall through the curved space precisely to our point of observation “P”. So we might mistakenly believe that we are observing views of a greater number of different objects. And the individual views are arranged in a ring in the sky, the diameter of which increases with increasing distance to the observed object.
Sketch 3 shows the limiting situation for the maximum distance V=2•R, when the star would be observed in the opposite direction in the apparent position “Z” and “Z´“, i.e. lying on the tangents “t” and “t´” which together form an angle of 180°. Sketches from 1 to 3 are created in the picture by continuously turning the circles “NK” and “NK´” around point P towards each other, until in sketch 3 they merge into one circle. By turning further, the situation would again change smoothly from sketch 3 to sketch 1, with the difference that the circles would be exchanged with each other.
In sketch 3, rotating the circle “NK=NK´” around the line PS would stretch and inflate the rugball to form a sphere of radius “R“. For a space with an absolutely constant curvature, we would again observe an illuminated circle created by the rotation of the “Z” and “Z´” positions around the line PS. And for a real space deformed by unevenly distributed gravity, the illuminated circle would again be torn to specific locations, from which the light would fall precisely at our point of observation “P” by curvature.
This model of self-contained space-time with spatially constant curvature applied to our looking into the Universe predicts the consequence that we cannot avoid observing individual objects in multiple directions at the same time. The individual views, which we can mistakenly consider to be different objects, are arranged in the sky in a ring, the diameter of which increases with the increasing distance to the observed object, and whose center lies in the direction of the P-Z line, where the observed object seems to be hiding from us in space-time.
In conclusion, the first suggestion is made as to how such objects or their groups that we observe twice or more in different directions could be searched for in the sky accordingly. But it is a much broader invitation than some failed attempts at the same thing in the past. If such multiple observations predicted by the model could be found in the Universe, then it would undoubtedly be an encouragement that even such a simple model could be useful for our insight into the space of the Universe as a whole.
