Authors: brilliant predecessors in my interpretation.
Composed by VVvv. Translated by Google translate, ran by VVvv.
Thanks are given to professors Jiri Bicak and Michal Krizek.
Version Tenerife – Miraverde, September 10, 2021
Translated October 29, 2021
This version is the first part of a series of articles created by dividing the original zero version “02NdV1_EN Looking into Universe, Part 1” into parts.This was done after consultation with Professor Michal Krizek from the Mathematical Institute of the Academy of Sciences of the Czech Republic, as an expert in cosmology and a member of the Cosmological Section of the Czech Astronomical Society and the Union of Czech Mathematicians and Physicists.I thank him for the valuable comments and opinions I have received from him.
The Abstract Abstract Looking into Universe.1 {2NdV.1A_EN} and Summary Looking into Universe.1 {2NdV.1S_EN} are available for this work.
(Notice to reader: The timestamp of the parentheses tells me when the note was either created or reformulated.To keep the versions organized, if I change the text or image then I change the date of version by at least one day.The whole words written in capital letters are words from dictionaries, which I use in a specific meaning, and which I am specifying in my writings.The original files are in Czech, my mother language.If there are discrepancies between the translations, the version in the original takes precedence.)
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20210101
I warn the reader in advance that the following compilation of my notes may not be entirely suitable for all “Main Stream” physicists.
From my studies in psychology, I realize that I’m reporting something here that some may not want to hear.
I came to this conclusion when the professor of theoretical physics at Charles University in Prague, Jiri Bicak, pointed out to me that he considered my description to be “parallel” to the description in ordinary physics.Or even more precisely, as he thinks, my description and the classical description are mutually skew. I WAS VERY LUCKY HERE, BECAUSE A FATEFUL COINCIDENCE CAUSED ME TO BECOME FRIENDS WITH PROFESSOR BICAK ALREADY IN MY CHILDHOOD.WE SPENT OUR HOLIDAYS IN THE SAME SMALL VILLAGE NEAR PRAGUE.
My first suspicion that a lot of things may not be as they are presented may have followed righty after the distribution of gifts by Jesus, angels, or Santa Claus.In the course of my life, some of them qualified from suspicion into working hypotheses, in which various alternatives slowly began to emerge, and became the food for my thinking, and with it also the related search1.
And when I failed to remove an alternative and replace it with a better one, even after a diligent effort, the unresolved alternatives began to automatically be stored in my INNER CONVICTION. And since this controls our actions, I had no choice but to start dealing with them.
At least I started offering them to smarter and/or wiser to remove them for me and help me replace them with better ones.
[1] I call these searches “Projects”. I have most of my projects in the field of psychology, which I never had the opportunity to study officially, so I caught it up with my whole life study. For example, how is it even possible what the statistics show us that even extreme alternative treatments [NL: Kwakzalverij] help to cure? Such as simply placing the healer’s hand on the patient’s ill place [NL: Handoplegging] can have such an effect? This led me to a project I called the “Placebo effect without placebo”, which I worked on for many years.
In the second place in terms of the number of my projects it is undoubtedly physics. This is probably because in philosophy I actually focused only on some genius thinkers, with whom I compared our current possibilities, our knowledge and our achievable consciousness. For example, the philosopher René Descartes did not yet realize why God had created him so that he could not understand all the truths of the world (he was created not to know everything), although he never blamed him or condemned him for it.
One of them is the center of my presentation, in which I try to edit my notes so that they at least somehow follow each other, and the reader has a chance to have his own opinion about them.I apologize in advance for the shortcomings in my description.I will also offer the reader a list of consequences, as I see them, and I hope that some of you will point out to me the shortcomings, possible mistakes, or at least some options to improve my description or replace it with a better IDEA. Or at least the reader will take something from it for his own use.
To start more specifically, I present here the following cut from https://en.wikipedia.org/wiki/Inflation_(cosmology):
“Overview
Main articles: Expansion of the universe and Inflation
Around 1930, Edwin Hubble discovered that light from remote galaxies was red shifted; the more remote, the more shifted. This was quickly interpreted as meaning galaxies were receding from Earth. If Earth is not in some special, privileged, central position in the universe, then it would mean all galaxies are moving apart, and the further away, the faster they are moving away. It is now understood that the universe is expanding, carrying the galaxies with it, and causing this observation. Many other observations agree, and also lead to the same conclusion. However, for many years it was not clear why or how the universe might be expanding, or what it might signify.”
Apparently, “Main Stream” physics was content with such a primitive IDEA in my eyes. After all, if we look at an object in the distance, we see it at the same time in the past. This cannot be otherwise, because information about that object flies to us at the speed of light. If we see an object, say, one million light-years away then we see it as it looked a million years ago.
And now beware: If we observe that all objects are moving away from us to a greater and greater distance then they are also moving away from us into a greater and greater past.
< 20200907
Should I observe how our space expands, where at greater and greater distances we see how the distance between the observed objects increases with the expansion, the gravity between them weakens [even with the square of their distance], so the space is somehow ” thinning” for us? Am I supposed to act like I’m in some “travel agency” looking at tempting landscapes farther and farther away?
Or should I observe how our space shrinks, when in the larger and larger past the distances between the observed objects were decreasing, the gravity between them was getting stronger [also with the square of their distance], so the space somehow “thickens” for us? Should I act like I´m in some kind of “museum” looking at older and older exhibits?
20200907 >
If I look spatially at objects receding in all directions, am I looking in the direction of expansion from the center I am in? And if I look at older and older versions of objects, am I looking into the past against the direction of expansion in time from the center I am in? What expansion are we talking about here? It just doesn’t work for me!
In the following, I will try to guide you through my first and so far only expedition to the distant Universe, which I managed to write better from my notes, so I dare to offer it to you.I will invite you to a kind of Gulliver’s journey to the world of giants, to the macro world, in order to somehow distinguish this journey from the description of the other two Gulliver’s journeys to the world of dwarfs, it is to the secrets of the micro world, which I also have prepared for you.
20210101 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20200726
Let us remember how long ago people were confronted with the IDEA that our Earth might not be flat, but could be round. I vaguely remember once reading some objections, such as “that it´s not even possible, because people on the other side of the Earth would fall into space”.And yet we had to accept the fact that the Earth is round. So ideally speaking, because the observable surface of the Earth shows us mountains and valleys, and the rotation of the Earth has also made it slightly oval, as an example that it cannot be ideally round.
20200726 >
< 20201201
Observing the Universe does not suggest to us that the Universe should have an end somewhere. But on the contrary, it offers us a slightly tempting IDEA that the Universe could even be unlimited, infinite. I don’t know who was the author of the idea that our Universe some closed space could be. I will venture to attribute this idea to Einstein, who promoted such an IDEA. But I should immediately apologize to everyone else and especially thank all those who deserved it. There may not be a single person who has received such an idea, and that it is actually an idea that has been born in many different places at once or in succession.
So, as a starting point, we will choose to search into closed spaces, maybe we will find a way how further. And if not, it can’t hurt. Anyway, at least we’ll find out what they offer us. In order for spaces to be closed, they must be curved. So let´s start the search.
But first, we simplify our task considerably by limiting it to curved spaces with constant curvature. Such spaces can be described mathematically and represented more specifically by geometric shapes, which somehow help us imagine such spaces. And they will also help us to be more easily aware of the consequences we are looking for. In order not to have to go into details here, I have compiled a separate article Mathematical Enclosed Spaces {0mup_EN}. It could help us to correctly understand the consequences to which they lead us. The article does not only describe the spaces, but mainly what we could observe in them, if we could get inside them, as observers.
20201201 >
< 20170104
But we must be well aware that the real space of the Universe cannot have a constant curvature. The curvature is caused by gravitational effects, and these are unevenly distributed in the Universe. On the contrary, we observe how objects with a large mass, and thus creating strong gravity, are separated from each other by free space, filled with vacuum and sparsely fragmented mass, which hardly contributes to the creation of gravity. Locally it could be a problem.
But if we realize the gigantic size of the Universe, fragmented into an innumerable number of galaxies, and each galaxy into a huge number of cosmic bodies, stars, then for such a whole, the assumption of a homogeneous mass distribution in the Universe does not have to be such a big limitation.
20170104 >
< 20210606
Just like an archaeologist working on an excavation somewhere in a deep canyon, the accuracy of the rounded surface of the Earth might appear completely different to an astronaut observing everything from outer space.
20210606 >
< 20201020
Before we begin, I would like to point out to you that an absolutely uniform, homogeneous distribution of matter and gravity in the Universe is not even possible. Such a Universe would be absolutely unstable. And I can state this with high certainty, because the study of instability has become part of my professional career. So instability becomes an integral part of my deeper study.
And this instability would cause the homogeneous distribution to immediately begin to tear and crumble into places of condensed matter that would begin to further compress, attract each other, move relative to each other, orbit each other, and rotate.
20201020 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20170104
One-dimensional closed space with constant curvature.
In the circle that represents such a 1D space, we can observe everything in only one direction forward/backward. Note that the circle has no beginning or end anywhere, so it has no center, only the center of curvature, which lies outside it. And every point of the circle is in contact with the space outside it, it is the space inside and outside the circle, as well as above and below the circle drawing plane. That is, with a space that actually already lies outside our investigated 1D space (for easier communication and clarity, I will use the term HERE for the investigated spaces to distinguish them from the spaces with which they touch at every point, but already lie outside it, and which I will called THERE). If we move around the circle long enough, we will return to the starting point but from the opposite side.
Let us realize that all our observations are, as it were, projected onto a plane perpendicular to the direction of observation, which I call the PLAIN OF OBSERVATION. And since we can generally do our observations in all different directions, we can replace this plane with what we call the OBSERVATION BUBBLE, which completely surrounds us as observers.
If we put our eye in 1D space, into the circle that represents it, then we would see only one point projected onto the surface of such a bubble in the direction of the tangent to the circle. We would not see the magnitude of the curvature, or at least in which direction it is pointing, we will not even see if there is any curvature at all, since the light propagates to us along the arc of this circle. We won´t actually see anything from the surrounding space THERE, into which we already have to put at least the center of curvature of the space.
And if such a space, for example, increases uniformly, then this would mean that after a certain time interval ∆t it will be represented by a circle of ∆R larger radius. However, the distance between fixed points on the circle does not increase by the change in the radius of the circle ∆R, but increases by the distance measured along the circular arc between them, as we would observe. Then, for example, the point on the opposite side of the circle to the point of the observer would move away by π·∆R, it is by the length of the arc corresponding to the difference in radii ∆R for the center angle π. And a point twice as far away, in our case lying back at the observer’s place, would be observed as if it had moved twice as far away, it is by 2π·∆R. If we also observe this change for the same time interval ∆t, the speed of the move away will appear to be twice as fast.
In general: for a uniform increase (decrease) of the space curvature (of radius R), the speed of receding (approaching) will appear to us to be directly proportional to the distance of the observed objects.
20170104 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20170104
Two-dimensional closed space with constant curvature.
The same applies to the surface of a sphere, as an example of such a 2D space. But there we can observe everything in all combinations of two mutually perpendicular directions, forward/backward and left/right. And notice again that the surface of the sphere has no beginning or end, so it has no center, only the center of curvature, which lies outside it. And every point of it is in contact with the space outside it, that is the space inside and outside the sphere.
If we maintain a straight direction while moving on the surface of the sphere, then our path will have the shape of a circle, which I call a SUBSTITUTIVE CIRCLE, because it substitutes our straight direction of movement on the surface of the sphere. And it will take us back to the starting point, but from the opposite side.
This will make it easier for us to realize what we would observe in such a space. By turning our head from left to right, the SUBSTITUTIVE CIRCLE would turn with us, and we would only see a straight line on our OBSERVATION BUBBLE. Again, we would not see anything from the curvature of space, if there is any at all.
And what would we observe if such a space enlarged uniformly? Since the SUBSTITUTIVE CIRCLE would also increase equally with it, the conclusion must also apply to it that the speed of receding (approaching) will appear to us to be directly proportional to the distance of the observed objects.
But what consequences should this have on our observations in 3D space? We will show that in the next chapter.
20170104 >
< 20210112
Often, as I have accompanied my fellow advisors/opponents along this well-trodden path of mine to here, it is at this point that our mutual misunderstandings have arisen. So that I don’t have to keep coming back to them, I will now try to explain what I think is at the heart of these misunderstandings.
And since they are basically two groups of mutual misunderstandings, I will start with the easier one for me. The first objection was, “Why can’t I observe the curvature of the surface of the sphere when I see ships disappearing beyond the horizon of the sea on the horizon?” This shows a misunderstanding of what we are able to observe from inside and from outside of this two-dimensional space that the surface of the sphere represents to us.
Yes, if we could leave this space, stand on the surface of the sphere, and observe its surface from some height, that is, from outside this space, then we would see how the ships disappear beyond the horizon as they move away from us, as if they were sinking into the sea. Conversely, ships moving towards us would begin to appear on the horizon, like submarines emerging from the sea.
No, if it is not possible to observe from outside, but only from inside, our eye will still see the light emitted from the ship as a point on our OBSERVATION BUBBLE. And since light can only propagate through that space (we have trapped it there after all! ) it will radiate towards us along the arc of the SUBSTITUTIVE CIRCLE, so we cannot observe any CHANGE in position (only a decrease in light intensity when ship is moving away, or an increase when approaching). We cannot observe any curvature, or even decide from our observation whether there is any curvature at all. Does the observed weakening or strengthening of the intensity of light come to us along an arc, or along a straight line which is this tangent to the circle?
I hope that I have succeeded in sufficiently clarifying the nature of the first group of misunderstandings, so we will proceed to the second group of objections: “Why can’t I decide whether I am on a plane or on the surface of a sphere?If, say, brown-eyed Eve and blue-eyed Adam on the globe set off straight from the pole in directions at 90° to each other, using navigation they would both maintain a straight course until they reach the equator, where they would both turn 90° towards each other and continue to each other, then they would meet halfway across the equator.They would thus complete a circular tour in the shape of a triangle, whose sum of angles on the surface of the sphere would be 3×90°= 270°. Whereas on a flat surface a closed path would
require turning along the 60° angles of an equilateral triangle, and their sum is only 3×60°= 180°?“
Yes, such an observation could be made by moving in such an enclosed space. In our example by moving 3 quadrants of the sphere´s surface long. But if we were able to move one more quadrant length, we could simplify the objection. Adam and Eve could travel from the pole together in the same direction along the meridian until they returned together to their starting pole from the opposite side. And that is clearly only possible on a sphere, but never on a flat surface. So that would also be clear evidence.
No, because if we could not move on the surface of the Earth, then we would not be able to distinguish the two closed paths (one on the surface of the sphere, the other in a plane) by observing from one point. If some light source were moving instead of us, from inside the space we would observe from the position at the pole only two fading points on our OBSERVATION BUBBLE, separated in our case by an angle π/2 (90°). And that movement along the equator would appear to us like as along a straight line between these points. We would not observe anything else. We would not observe whether the light sources were moving along the curved surface of the sphere, or in a tangent plane to the surface of the sphere at the point of observation. We wouldn’t have any idea whether this plane of our observation is a tangent plane to any sphere at all.
I hope that with such an additional more detailed description, we can more easily imagine observations inside curved spaces.
20210112 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20201201
For 3D open spaces, it is common to use the Cartesian x-y-z coordinates to describe them. But this does not seem to me to be advantageous for closed spaces. Far more preferable is the description using spherical coordinates r(≡z=c·t, where c is the speed of light)-φ-ψ centered at the observer’s location. We can observe in directions that are any combination of the angles φ and ψ and thus directions left/right and up/down, and when moving we can add a third direction, forward/backward.
But how can we imagine such observations? And how can we even imagine such a 3D enclosed space? It is no longer possible to help us with geometric shapes known from open spaces. And our CONSCIOUS THINKING may not even be ready for such IMAGES. Then trying to create them could be a waste of time. However, when moving through such a space in any combination of all possible directions should again lead us to the original place from the opposite side, we can again advantageously use our SUBSTITUTIVE CIRCLES to describe the consequences for our observations.
20201201 >
< 20200712
I will offer you a visualization attempt with a sketch that I called SPACE 4D [2P4D_EN]. Why four-dimensional? Let’s realize that the circle is a two-dimensional self-enclosed figure, which represents to us a space enclosed in itself in such a way that it only appears to us as a one-dimensional space from inside (and beware: it even appears to us as a one-dimensional non-curved space, which we can no longer distinguish from curved space).
Similar to how the surface of a sphere is a three-dimensional self-contained surface, which represents a space that appears to us as two-dimensional when observing from inside.
And so now we are basically dealing with some four-dimensional self-contained formation that represents our space in such a way that from the inside it just appears to us as a three-dimensional uncurved space.
But where are they and what are the four dimensions? In addition to the three “spatial” coordinates x, y and z, we have another “temporal” coordinate t (hence such a space is sometimes called “space-time”). And here, in our IMAGINATION, in our model, we connect the time coordinate t with the space coordinate z by the relation z= c·t, where c denotes the speed of light propagation in a vacuum1. Yes, we marked it in red to stand out really well. Why? We will clarify that right away.
In Newton’s time, we had time running exactly evenly, better than clocks. And the space didn’t change for us, no matter what we did. But under Lorentz and Einstein, who laid the foundations of a new IDEA, the new field of physics called Relativity3, it all fell apart.
We started to slow down time, and in some places with a high concentration of gravity called Black Holes, we even stopped time completely!
And what did we do with the dimensions of the space? We also started to shorten them, and in that singularity called a Black Hole, even to zero! Yes, we are really able to do that, at least in our IMAGINATION.
[2] < 20210223 In order to follow the sequence of geometric shapes with the initial letter “k” in the Czech language (1D: “circle”, 2D: “sphere” [CZ original: 1D: “kružnice”, 2D: “koule”]) introduced in Mathematical Enclosed Spaces {0mup_EN}, I would like to call a geometric shape that would represent a closed 3D space with constant curvature by “KOZAK”. The name was somehow created by shortening the Czech words for “constant curved shape”.
Although of course I cannot imagine its geometric appearance, its mathematical description should be such that it expresses analogously its “volume”, let´s say Ψ, in units [m4]. This is because the “volume” that the circle encloses, which we call the circle area comes out in [m2], and the volume of the sphere in [m3].
And just as a circle represents 1D space to us, and the surface of a sphere represents 2D space, then the surface of the KOZAK form would represent 3D space.
To somehow deal with the IDEA of “space-time” that contains 3 spatial coordinates and one time coordinate, I bind the temporal form to the spatial one using the speed of light propagation c. Spatially it will remain as ΨL[m4], but temporally it will be bound to ΨT[m3•s] by the relation: ΨL[m4] = c[m/s]•ΨT[m3•s]. 20210223 >
[3] < 20210121 Relativity is a mathematical concept that has become an accepted model. Currently, a large group of scientists consider the concept proven, another group unproven.
Expecting some concept to be provable is like expecting nature to start following some mathematical concepts of ours. But the opposite is true: Our models describe some part of the observed natural phenomena well, while others do not. Often the new model describes at least better and/or more phenomena than earlier models.
It will probably be as someone put it in the mouth of Stephen Hawking, that “he who ever proves the truth of the theory of relativity really deserves the Nobel Prize.”
(I formulated this note just on the 21st day, 21st year, 21st century) 20210121 >
And what is left to us in that space unchangeable? Just the last constant c. But not in the honorable last place, but on the contrary in the first place when seen from the opposite side 🙂

Let´s choose one place of our observation and one direction of our observation, for example in the direction of coordinate z, as shown in the sketch. Then our observation in such a constantly curved space with a radius of curvature R is along the arc of SUBSTITUTIVE CIRCLES. One such is drawn, where the direction of our observation forward along the arc of this circle has the coordinate z=R·φ, while we leave other coordinates perpendicular to it to the right and up straight, not curved, like coordinate axes x and y.
So the origin of our observation lies in the x-y plane. But our entire observation lies in the x-z plane (indicated by horizontal hatching in the sketch) called the ρ plane. So our observation along the arc in the figure “runs” from the straight line to the left in the direction of the negative x-axis. If we were to move along the SUBSTITUTIVE CIRCLE in the z direction for long enough, we would get back to our starting point but from the opposite side. For the angle φ=2π, the arc would be closed in the whole circle.
It is clearly visible in the picture that the light that would propagate to us along the marked arc can no longer be distinguished by our eye from the light that would propagate to us along the marked tangent to the arc. And this is exactly why we can never directly observe the curvature of spaces from the inside.
But let´s be aware that in the given direction of observation we can lead a huge number of such SUBSTITUTIVE CIRCLES, which we would obtain by rotating the plane ρ of the drawn SUBSTITUTIVE CIRCLE around the direction of observation. The centers C of such SUBSTITUTIVE CIRCLES would describe the arc marked in blue. And at the beginning of our observation, the SUBSTITUTIVE CIRCLE would form a kind of rotationally symmetrical “cone” on the outside, also faintly colored in the picture (the cone is drawn as if bounded by a circle, which is created by rotating the white marked point on the SUBSTITUTIVE CIRCLE). And inside the SUBSTITUTIVE CIRCLES, a shape would be created that would be the shape of a tire tube with zero opening inside. That is, a kind of children’s swimming ring with a zero opening for a child. The name “annulus” is often used for such a geometric shape, and I will therefore call it that.
I cannot imagine the 4D form of a self-enclosed space with constant curvature as a whole, but both the “cone” and “annulus” just described shapes become imaginable for us in the given direction of observation. But let´s realize that such shapes should be observable in any direction of observation.
For an ideal space of constant curvature, the probability of our view and/or movement would be absolutely equal for all SUBSTITUTIVE CIRCLES formed in our direction of observation. As we will show elsewhere, in real space our actual observation and/or actual movement forward would be reduced to one single circle. The sum of the probabilities of the occurrence of individual circles, which would mean certainty, would “collapse” (as this phenomenon is called in physics) into one probability, into the certainty that would affect us.
20200712 >
< 20210418
Let’s try to imagine it in a space one unit smaller. It´s as if we were standing on the surface of the (Earth-)sphere and thinking about which direction we should go on our journey, when all directions would be equally valued for us. And only after taking a step would all the same probabilities of directions “collapse” into the probability of one, into the certainty of the direction in which we set out on our journey.
20210418 >
< 20200912
By imagining an observation in 3D space with constant curvature as observing along a SUBSTITUTIVE CIRCLE, we can express the observed distance from us as z=R·φ, where R is the radius of curvature of the space [radius of the SUBSTITUTIVE CIRCLE] and φ is the distance measured in arc measure along the arc of a circle with the origin at our point of observation.
And the speed of moving away (or approaching) fixed points on the circle (φ is constant for fixed points), which radius R increases (or decreases), we can then write as the time change of the distance along this circle, Δz/Δt= ΔR/Δt·φ, and for infinitesimally small changes Δ then as dz/dt= dR/dt·φ. Or we can also write that speed as ΔV= ΔV0·φ, when we mark the expansion speed dR/dt with the symbol ΔV0.
20200912 >
< 20210418
In the figure SPACE 4D [2P4D_EN] only one SUBSTITUTIVE CIRCLE is drawn from all such circles that would be formed by rotating the center C around the direction of observation as shown by the circle drawn in blue. By rotating through an angle of 180°, this circle would again intersect our plane of observation ρ at the point C´, as shown in the figure SPACE 4D in Cross section [2P4DvP_EN]:
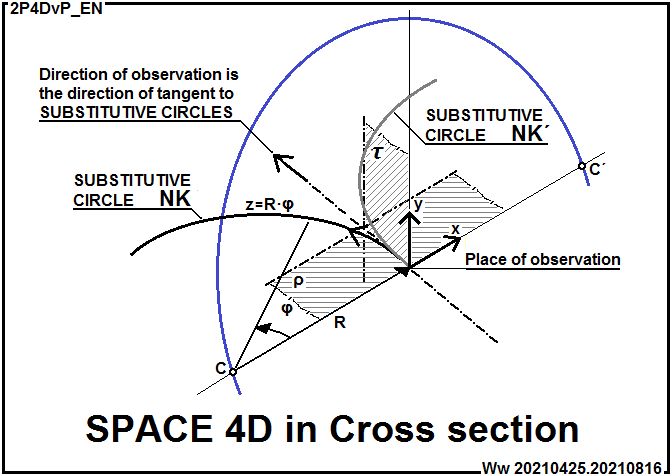
Our plane of observation ρ has become a cross-section of space in which the SUBSTITUTIVE CIRCLE NK (marked in black) has been joined by a second SUBSTITUTIVE CIRCLE NK´ (gray), which is symmetrical with respect to the plane of symmetry (indicated by oblique hatching in the sketch) called the plane τ.
20210418 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
< 20210511
In the previous chapter, we made a more specific IDEA of looking into a 4D closed space with constant curvature in the chosen direction. Let us now describe the consequence of observing a certain object.
The figure SPACE 4D in Cross-section [2P4DvP_EN] actually shows one special case, if the observed object were so close to us observers that the curvature of space would not play any role. As if the consequence of the curvature of space was not yet observable at such a short distance. Then the observed object would appear to us as if it lay at the intersection of the planes ρ and τ, it is in the direction of the tangent to the SUBSTITUTIVE CIRCLES.
In the picture STARS and LIGHT PROPAGATION G [2phG_EN], which actually complements the series of pictures from my article Observing Stars {2ph_EN}, the situation in section ρ is drawn as sketch 1:
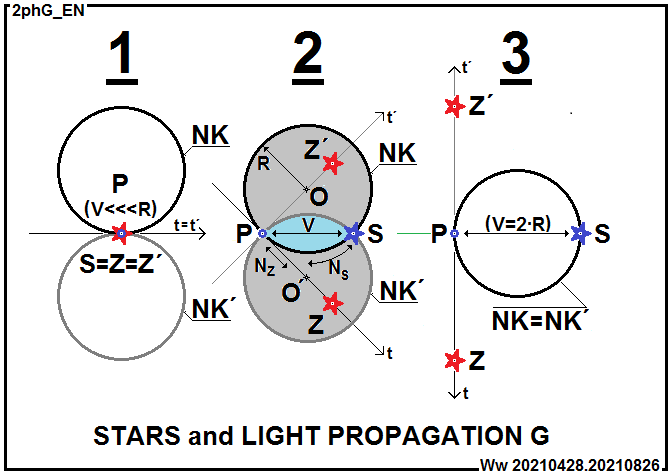
It is a situation where the direction of observation is drawn from left to right and the observed star in the actual position “S” is observed by us from the place “P” at such a small distance “V“ (V<<<R) that it would practically lie on a common tangent “t=t´“ to SUBSTITUTIVE CIRCLES “NK” and “NK´“.
For larger distances “V“, the situation is drawn on sketch 2 for which the situation is chosen, where the tangents “t” and “t´” to the SUBSTITUTIVE CIRCLES “NK” and “NK´” form a right angle of 90° together. But the observer from the point “P” observes the star along the tangents in the apparent positions “Z” and “Z´“.
Since we chose the section ρ arbitrarily, by rotating the section we would observe more and more pairs of apparent positions of the star, until for a space with absolutely constant curvature and sufficiently fine rotation we would observe an illuminated circle formed by rotating the “Z” and “Z´” positions around the P-S junction. The common enclosed space created by the rotation of the circles would be a rotationally symmetrical shape resembling a pointed rugby ball with a constant curvature. So a kind of light blue marked rugby ball, which we shall therefore abbreviate simply to “rugball“
Sketch 3 shows the limiting situation for the maximum distance V=2·R, when the star would be observed in the opposite direction in the apparent position “Z” and “Z´“, it is lying on the tangents “t” and “t´” separated by an angle 180°. Sketches from 1 to 3 are created in the picture by smoothly rotating the circles “NK” and “NK´” around the point P towards each other, until in sketch 3 they merge into one circle. With further rotation, the situation would again change smoothly from sketch 3 to sketch 1, with the difference that the circles would be exchanged with each other.
[4] < 20210611 For completeness:
By turning the circle around the skew line, a formation called “torus” is created (a kind of children’s swimming ring with a hole for a child). If the skew line approaches to touch the circle so that it becomes a tangent of the circle then rotating the circle around the tangent will create a shape that we call “annulus” (a kind of children’s swimming ring without a hole for a child). And by turning the circle around the secant, an inner form called “rugball” is produced (marked in light blue in the picture) and outer form resembling a wreath, or better a “paraguayo” [Paraguayan peach] (marked in light gray).
By rotating the SUBSTITUTIVE CIRCLES in the picture around point P, going back from sketch “3” to sketch “1“, the inner rugball shortens and flattens, but the outer paraguayo grows larger until it turns into an annulus and the rugball disappears. Along the tangent to the SUBSTITUTIVE CIRCLE, light propagates towards us from both sides. We can observe every object on the circle from both sides. We observe not only the front side closer to us and thus the younger version along the surface of the inner rugball, but also the back side further away from us and thus the older version along the surface of the paraguayo. 20210611 >
In sketch 3, rotating the circle “NK=NK´” around the P-S line would stretch and inflate the rugball into a “sphere” of radius “R“. For a space with an absolutely constant curvature, we would again observe an illuminated circle created by rotating the “Z” and “Z´” positions around the P-S line.
20210511 >
< 20210904
I got an idea how I could help readers understand the situation more precisely:
Imagine a situation where light would respect the curvature of a perfectly round Earth, so that it would only propagate in a straight direction along its surface. And we would stand at one pole and watch the light turn on at the opposite pole. Along which meridian would we see the light coming?
Yes, that’s right, along all meridians.
And that, translated into our space-time model, is exactly what the limit situation in sketch 3 shows us.
20210904 >
< 20210513
And in real space, which cannot be absolutely constantly curved, because the unevenly distributed gravity has deformed it into a space with a locally fluctuating magnitude of curvature, the illuminated circles would tear for us into specific locations, from which the light would fall in a curve precisely to our point of observation “P“. The model thus predicts the consequence that we cannot avoid observing individual objects in multiple directions at the same time. Individual views, which can be mistaken for different objects, are arranged in a ring in the sky, the diameter of which increases with increasing distance to the observed object. The effect looks like a global gravitational lens due to the weak global gravity that holds the Universe together, and resembles local gravitational lenses, an effect that is already known from observations of strong local gravity around Black Holes.
20210513 >
2NdV.1_EN Looking into Universe.1
A. INTRODUCTION
B. STARTING POINT
C. 1D SPACE WITH CONSTANT CURVATURE
D. 2D SPACE WITH CONSTANT CURVATURE
E. 3D OR 4D SPACE?
F. OBSERVATION IN CURVED SPACE
G. POSSIBILITY OF MODEL VERIFICATION
With thanks, I remember what Professor Bicak told me during one consultation: “A working hypothesis becomes useful when it predicts something that can be verified by observation”.
< 20210210
And in my opinion, this is where we have the opportunity to verify this model. Verification, whether such a highly simplified model, which I call the Einstein model here, namely the mathematical model of a closed space with constant curvature, could be used to roughly describe the real space-time of the Universe as we observe it as a whole.
I will point out such a possibility using the picture STARS and LIGHT PROPAGATION B [2phB_EN], which I will borrow from my article Observing Stars {2ph_EN} and supplement it under the title Consequences of Looking into Universe [2DNdV_EN].
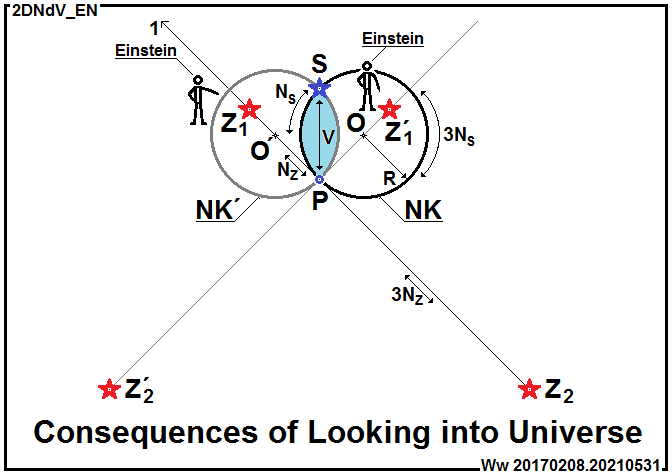
20210210 >
To explain it, I copy from that article:
< 20170208
Let´s imagine that the plane of our paper is an arbitrarily chosen plane that intersects our space in such a way that one star that we will observe lies in it, and that our observer´s eye also lies in it. The picture depicts such a situation where our eye at point P will observe a blue star at point S.
In a plane, we can fit only two circles of radius R through two points that are virtually separated by a distance V, where 0<V<2R. Why “virtually” distant? Because in a space with constant curvature, we could only move in circles and make our observations only in circles. The line along which we measure the shortest distance between points P and S in the figure, here as V long, lies outside this space. That is, it does not lie in the space that we investigate and call HERE, but it lies entirely in the space called THERE.
One SUBSTITUTIVE CIRCLE NK in the picture is drawn fully, the other NK´ more weakly. Both circles are drawn for the selected distance V = R·√2, so both circles will intersect each other perpendicularly. In other words, the angle φ between the tangents at the point P to the circles with their center of curvature O and O´, respectively, will be true 90°. For this situation, the shortest length “NS” between points P and S, measured in the curved space HERE along the arc of the SUBSTITUTIVE CIRCLE, will be equal to a quarter of the circumference of the whole circle, expressed for example in number of light years, as NS = R·π/2.
The light from this star will then fall into our eye from the direction of the tangent to the SUBSTITUTIVE CIRCLE NK at the observer´s place, marked by the arrow “1“. The star will appear to us in the apparent position “Z1” distant from us NZ = NS light years. But the light from the same star will also propagate towards us in the opposite direction along this SUBSTITUTIVE CIRCLE NK, it is along an arc “3NS” long, and will appear to us in the apparent position “Z2” 3NZ = 3NS light years away from us. If we don´t block the light that was propagating towards us, then that light could go around the entire SUBSTITUTIVE CIRCLE one more time, or more, and thus we would observe it in the same direction of tangent 1 but from distances of 5NZ, 7NZ, etc.
The situation of light propagation along the second SUBSTITUTIVE CIRCLE NK´ will be similar, and will lead to the observation of the star in the apparent positions “Z´1” and “Z´2“, indicated in the figure.
But we inserted the plane of paper into the space arbitrarily, so we could also insert it there in a slightly rotated position along the axis passing through the points P and S. This would lead to other apparent observed positions. But by gently rotating the plane of the paper, we could make countless such positions. This would have to lead to the conclusion that in an enclosed space with absolutely constant curvature, we would no longer see a single star, but an illuminated ring centered at P-S, from which light from S would radiate to us in NS years. And also from a ring nine times dimmer, from which light would radiate to us in 3NS years and from others in 5NS, 7NS, etc.
20170208 >
< 20210210
Unevenly distributed gravity tears such an illuminated ring into individual points of light, which easily tempt us to mistake them for various different observed objects.
When light propagates from them to us in the vicinity of strong gravity, the light´s path is additionally bent depending on the intensity of gravity. As a result, we observe objects slightly displaced from their positions. The closer the trajectory is to the center of gravity, the stronger.
It reminds us of the effect known from geometric optics, where lenses bend individual light rays depending on their distance to the center of the lens. But conversely, the further they pass from the center of the lens, the stronger.
Verification of the expected consequences of the model would basically consist of searching for identifiable objects or, in general, entire groups of objects that we would observe in the sky twice or more times in different directions. But it would still be two or more observations of just the same identified group of objects. The proposed verification option consists of:
(1) Searching for such groups of objects that we observe in the sky several times in different directions
And Einstein shows us just an example in the picture as two red-marked observations Z1 and Z1´ of one blue-marked star S.
They would therefore be observations of one and the same group, which we would observe along the SUBSTITUTIVE CIRCLES as being equally distant from us in space and time. But we would observe such a group in the sky twice or more times in different directions. Those directions would form the surface of a cone (the opening angle of the cone is π/2 in our figure). And precisely these directions, together with the observed red shifts of light, would determine the corresponding position R·φ of the observed group in the space of the Universe.
20210210 >
< 20210511
That is, the search for groups from which light radiates towards us, through the surface of a geometric shape called “rugball“, marked in light blue in the pictures. But it propagates through its surface, deformed by the uneven distribution of gravity, so we observe the incident light torn, as if it were radiating to us from discrete points in the sky.
20210511 >
< 20210202
But since we observe objects in curved space along a circular arc, then the farthest observed object can never lie further than on the opposite side of the circle, it is at a distance φ= π from the observer. Because any observed object for φ> π would actually already be observed in the opposite direction for φ <π. However, we cannot include such an object in the Universe twice, because it cannot even be in the Universe twice. In order not to count the space of the Universe in the “second round”, then the “real” SIZE OF THE UNIVERSE would come out as Rv= R·π.
In other words, for φ= π =180° (when the rugball formation would transform into a sphere, and the two circles in the figure would merge), the length of the arc R·π would determine the distance of the observed object from us, which is just at the distance called SIZE OF THE UNIVERSE Rv= R·π. That is, in a space that we do not count for the second time or as if in the “second round”.
20210210 >
< 20210112
For a space one size smaller, it would be like slowly moving along the surface of the Earth in the same direction until we spatially return to the place from which we started. But at the same time we would return to the same place time-shifted. Sure, a lot of time has passed since then, quite possibly old buildings or other structures have been rebuilt, or demolished and replaced by other structures. It is quite possible that we wouldn´t even recognize the starting place anymore.
In any case, there is a risk that we start to count some part of the space we observe twice, maybe even more times. The worst part is actually the question, what is the Universe right now, the space of which we are counting? After all, what we see, for example, at a distance of a million light-years, is only what was there a million years ago. But what is there now? We could see that in a million years, but neither you nor I will be here (so it shouldn’t worry us so much 🙂 ).
20210112 >
I have received a notification from Professor Krizek, for which I thank him. Attention paid to unsuccessful attempts for such a search that has already been realized.
< 20210425
I understood from them that it was a matter of comparing the spectra of the emitted radiation that characterizes each galaxy, by observing in two opposite directions, it is in directions of observation that form an angle of 180°.
I can imagine that each galaxy has a unique distribution of masses and distribution of velocities with which they orbit a common center of gravity. And I can also imagine that the middle plane of the galaxy will be uniquely tilted towards us observers. Then the individual contributions of the orbiting masses to the red-shift will also be different as a result of their moving away from or approaching us observers. The so-called Doppler Effect is to blame. The Doppler Effect or principle is popularly known to the general public in acoustics. For example, the noise of approaching cars, be it the sound of an engine, siren or squealing tires, has a higher frequency that drops as the car passes to an audible lower frequency as the car recedes.
Such an optical effect apparently creates different spectra typical for each galaxy and for its angle of the plane of rotation with respect to our observation, as I understood from his explanation.
However, I would consider the chosen mutual observation angle of 180° in such experiments to be a strong limitation in the search for possible objects in the sky. And I would also consider any direct comparison of the spectra of light emitted by galaxies in past experiments to be a serious limitation.
The 180° angle does remove the problem of the angle at which we observe the plane of orbit (this plane is equally inclined to us observers in both directions of observation). However, due to the rotation of the galaxy and the moving away and approaching of individual masses, the local Doppler contributions in both such observations are exactly opposite to each other. The local contribution, which appears to us as receding in one direction of observation, appears as approaching in the opposite direction (and vice versa).
An exception would be a galaxy that would have its plane of rotation exactly perpendicular to our observation. There, the Doppler contributions due to the rotation of the galaxy would disappear from our observation in both directions, so that the spectra of emitted light observed in both directions could be directly correlated. However, finding such a galaxy could be an amazingly large coincidence. Therefore, I would consider this a complicating and limiting factor of such verification attempts.
But I now call for a broader comparison:
For example, objects that are much closer to each other than the radius of curvature of space R can be considered locally as in 3D non-curved space. Then, by rotating some arrangement of such objects, some view of it might just correspond to the distribution of the objects we observe in the sky in different directions. And this could be the indication that we observe one arrangement in the Universe twice (or more times) just in different directions.
Or, for example, let´s take two cosmic objects that orbit each other around a common center of gravity in such a way that they overlap with respect to us observers, and thus during one orbit we observe fluctuations in the intensity of radiation. Then we could also observe fluctuations in the intensity of radiation with the same frequency at another place in the sky, but phase-shifted from each other. And that phase shift would determine the angle between the two observations.
Of course, finding even such situations in such a huge number of observed objects could be a rare coincidence. But the one who succeeded in such a thing would become world famous, so he would be appreciated for his effort. And maybe that’s why there might be those who would like to try it.
And if the red shift for the observed object corresponded to each other in both directions, then it would also mean the same speed of receding and the same distance from us in both directions of observation. This would be because our corresponding spatial and temporal distance from such an object as observed or measured along SUBSTITUTIVE CIRCLES would be the same for both views according to our model of constantly curved space.
I see several significant differences between the proposed search and the mentioned attempts:
(1) First of all, the proposed comparison is not limited to the angle of mutual observations of 180°, but uses all angles from 0 to 180° (!) corresponding to the distance of 0<V≤2R, as shown by my suggestive picture under the title Consequences of Looking into Universe [2DNdV_EN]. There, the pair of observations Z1 and Z´1 (or Z2 and Z´2) corresponds to one the same object S.
I hope it is clear from the picture that the distance of star “S” from us observers in “P” measured along the arc of both SUBSTITUTIVE CIRCLES NK and NK´ is the same for each pair in the presented model (in the picture the shortest distance is equal to NZ=NS light years). And this is true for all “V” distances and thus for all angles between the two directions of observation (between the tangents to the SUBSTITUTIVE CIRCLE at the point of observation).
(2) And secondly, such verification does not depend on any particular IDEA of how or why observable cosmic objects move away from us. The verification is valid for SUBSTITUTIVE CIRCLES, which may or may not increase in size over time. This is because even if the radii of our SUBSTITUTIVE CIRCLES increased over time, the distances measured along the arc of such circles in the observation directions would remain mutually the same. The size of “V” would increase and the corresponding distances measured along the arc of the SUBSTITUTIVE CIRCLES “NK” and “NK´” would also increase, but they would remain mutually the same. This is because, in our model of space with constant curvature, the growth rate of the circle radii would have to be the same everywhere, and our observations in either direction would still have the same value. Only in this way would the model of space retain its property of constant curvature. We will return to the details of our observation in expanding space in the second and third parts of this document.
(3) And it follows from the symmetry of the SUBSTITUTIVE CIRCLES “NK” and “NK´” that the object observed in both directions appears to us to be equally distant and of the same age in space-time, so that even the red shift of light observed in both directions should be the same. And that might make our search easier.
I hope there might be someone enthusiastic about such a search. Perhaps he himself would realize that it would be in his OWN INTEREST. If he is lucky enough to be the first to find such objects, he will become famous.
20210425 >
To make it easier to understand the IDEA I´m describing here, I often helped myself by describing how it would look in a space one unit smaller.That is, in a self-contained space with constant curvature, such as the surface of a sphere.In the end, I will therefore try to compile such a description.
< 20210430
What would my challenge correspond to in a space one unit smaller than our space represented by the shape I call KOZAK, it is a 4D shape enclosed in itself with constant curvature so that its surface appears to us as a 3D space?
Such a space one spatial dimension smaller would be 3D enclosed within itself with constant curvature, which appears to us as 2D, it is one that represents the surface of a sphere.
Let´s imagine that even the Earth could be represented by an ideal sphere for this purpose. And let´s imagine that we were to investigate how we would travel along it from the observer point “P“, which would be my hometown Prague, for example, and we would like to travel to some point of interest “Z“, which would be let us say Rome.
The shortest path would be to pierce the Earth with the straight line “P-Z“, but it would lie entirely outside our investigated space, it is outside the surface of the sphere. Good. But let’s cut the spheres so that the connection “P-Z” is precisely the symmetry of the section, as shown by the suggestive sketch “1” in the figure Cross-section of Sphere [0PK_EN].
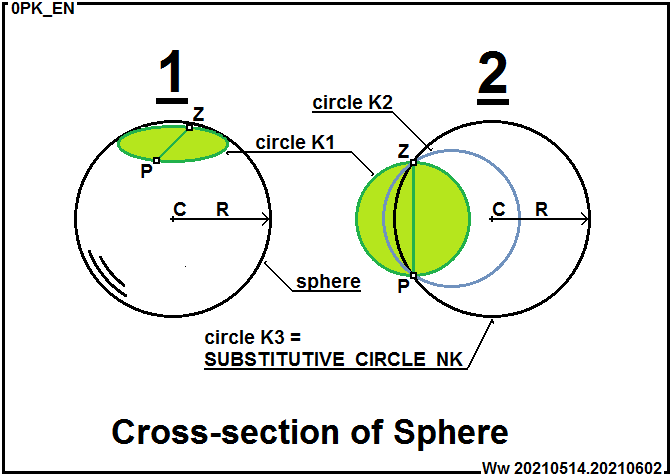
However, the cut marked in faint green on the sketch will cut out two equally long paths, equally curved arcs, symmetrical to each other on the surface of the sphere, as shown by circle “K1” on sketch “2“. And on the surface of the (Earth) sphere, both such paths will appear to us as curved but real, because they will only lead through places that all lie on the surface of the sphere, it is they lie in our investigated space. In doing so, they correspond exactly to what I suggest.
And what would it look like if we rotated the section of the sphere around the connecting line “P-Z“? Then this connecting line would no longer be the axis of symmetry of the section. That cut would again create two equally curved paths on the surface of the sphere, which would lie entirely on the surface of the sphere, but they would no longer be of the same length, as shown by the pale blue circle “K2” in the sketch. And this would apply to any rotation of the cut.
But the shortest arc (and the longest one corresponding to it) would be created by rotating the section around the connection line “P-Z” so that the section simultaneously passes through the center of curvature “C“, it is passes through the center of the sphere of radius “R“.
Such a “K3” circle would be the longest of all, representing the circumference of a sphere, just as the equator and meridians represent the circumference of the Earth. I call such a circle “NK” a SUBSTITUTIVE CIRCLE, because it substitutes the straight direction in the 2D space of the sphere´s surface. Just as it substitutes the straight direction in 3D space with constant curvature, the direction in which light would propagate in it.
And if our intention was to travel to any other point “Z” on the globe, we could always cut the sphere in such a way that the line “P-Z” would again be the axis of symmetry. And if the connecting line “P-Z” no longer passes through the center “C“, we could create new and new different circles by rotating the section around this connecting line, even create any number of them, if it is possible to refine the angle of rotation of the section for any length of time. But only one of them, made by the cut that passes through the center “C“, would be the SUBSTITUTIVE CIRCLE.
So what would be different about looking into such a 3D curved space that appears to us as a 2D non-curved space?
First, even if we were to travel from point “P” to point “Z” however fast, we would never travel at the limiting speed c that light radiates towards us through space.
Second, the number of circles that we could draw between the points “P, Z”, as in the sketch are “K1“, “K2” or “K3“, in our 4D curved space, which appears to us as non-curved 3D space, is “one dimension” more. And we select from them only those whose radius is exactly equal to the radius “R” of the curvature of space, and which we then call SUBSTITUTIVE CIRCLES. These correspond to the section of the (Earth) sphere that passes through the center of the (Earth) sphere.
But instead of one SUBSTITUTIVE CIRCLE, we could create an unlimited number of SUBSTITUTIVE CIRCLES for the selected “P–Z” connecting line in the 4D curved space by infinitely refining the angle of rotation of the section. Just like in the picture STARS and LIGHT PROPAGATION G [2phG_EN] SUBSTITUTIVE CIRCLES rotating around the P-S connecting line formed the surface of a light blue drawn shape called a rugball.
20210430 >
< 20210529
The introduction of the term “rugball” I hope gives me the opportunity to describe geometrically better the IDEA of what search I am calling for. The rugball, which is highlighted in light blue in the picture STARS and LIGHT PROPAGATION G [2phG_EN] and Consequences of Looking into Universe [2DNdV_EN], has the geometric form of a rugby ball with a constant curvature equal to the curvature of space, in one tip P of which we are the observers, and in the other S is the actual position of the observed object. However, light according to this model propagates along its surface from S to P.
It means that if we choose an identifiable structure or phenomenon that we observe in its apparent position, for example Z in sketch 2 in [2phG_EN], and which we want to search for in another apparent position, we still do not know where its real position S is, where the axis is of our rugball. After all, we can look for it in the sky in all positions created by turning the rugball around the tangent t., it is around the P-Z direction. So how we can proceed?
However, a simplifying factor would be if we knew a priori thus in advance at which angle φ to look for the turned rugball. Then the light under this observation angle would radiate over its surface and fall symmetrically into our eye under the same angle φ. All possible search positions would have to be searched along the cone, created by rotating the tangent t around the P-S axis, which would form a ring in the sky. But we still don’t know in which direction the actual P-S axis is? So we don’t know where in the sky is the center of such a ring? This would mean searching along all such rings formed by carefully rotating their centers around the tangent t.
A similar simplification would be if we at least roughly knew in advance which curvature R in our model best corresponds to our observation of the object in the Universe. Then, from the red-shift of the light and from its corresponding speed with which the observed object recedes from the Earth, we could determine the distance NZ of our observation. The model would then also show its distance measured along the arc NS=NZ, so we could also reveal the angle φ and thus the radius of all those rings that would determine the possible directions of further search positions in the sky.
What else does this imply? If we do not know a priori anything about the chosen object in its apparent position of observation Z, the possibilities of finding a corresponding other apparent position of it will be almost unlimited.
< 20210604
But if such a second apparent position Z2 was found in the sky, only two rings in the sky would have been determined, where to continue the search. They would be rings in the sky symmetrically placed around the connecting line of the Z and Z2 positions. And our next search would have to focus on them. By the time the third apparent position Z3 was found, there would be only one single ring left in the sky to continue the search. The position of the center of the ring in the sky would be known, the direction of the P-S axis of the rugball would be determined.
20210604 >
In connection with the already performed attempts of such a search, which Professor Krizek brought to my attention, I would like to describe such an attempt from the point of view of our model. That is, the search for an object that we would a priori assume is the farthest from us, that is, that it lies at the exact opposite end of the Universe relative to our position in it. It is a search for an object that is just opposite from us on the SUBSTITUTIVE CIRCLE.
This apparently led to the comparison of two observations always made in opposite directions, which form an angle of 180°. However, this would mean in our model that we already know a priori that the actual position of the searched object S lies exactly perpendicular to such direction t as in sketch 3 in [2phG_EN]. So all possible apparent positions of such an object lie on the ring formed by rotating the point Z (or the tangent t) around the P-S axis. Therefore, it is not enough to compare only the position at the opposite place 180° away, but all positions on that ring (!!!).
20210529 >
This concludes the description of the first part, the first stage of the search called Looking into Universe.In the next stages, I will present a compilation of my notes to describe further implications of using a closed-space model with constant curvature to roughly describe the space of the Universe as a whole.
I hope that the readers will forgive me for the inaccuracies in my description, and at least they will make their own IDEA about the subject.And those attentive readers will understand what I mean by the challenge to verify the predicted consequence of the model by skilled experts.I am curious about your and their reactions.
< 20210607
The motivation for all this effort was the unsatisfactory interpretation of galaxies receding from us, the more distant the faster, derived from the discovery of the red shift of the light they emit. In the beginning, we came out of admiration for the contribution to our civilization made by the discovery that we do not live on a flat earth, but on the closed round surface of the Earth. And the elegance of the idea that our entire Universe could be some limited enclosed space caused my search for IDEAS of enclosed spaces combined with my curiosity of where they would take us.
By separating the first stage into a separate part, we did not need any knowledge of physics yet. Using only our innate imagination, simple arithmetic and geometry, we worked our way up to discovering the first implications of a simple model of curved space with constant curvature. That it is no longer possible to avoid multiple simultaneous observations of objects from different sides. Individual views, which can be mistaken for different objects, are arranged in a ring in the sky, the diameter of which increases with increasing distance to the observed object. And even independently of any interpretation of the observation that objects are receding from us, and space is thereby expanding. Any expansion of space with constant curvature already inherently carries our observation of the more distant objects, the faster they recede.
If the multiple observations that the model predicts could be found in the Universe, then it would undoubtedly be encouraging that even such a simple model could be useful for our IDEA of the Universe as a whole. A kind of view from above, as a cosmonaut would observe it as a whole, would thereby be added to the ant efforts of cosmologists as archaeologists searching in the local details of the history of the Universe.
Let us now look together at other implications of the model of closed spaces. In the next stage, in the second part of Looking into Universe.2 {2NdV.2_EN}, we will search for the consequences that the model of a closed expanding space entails. So let´s start the search.
20210607 >
< 20210330
Acknowledgement
I thank all the brilliant predecessors, the authors of this work, who gave me the opportunity to try to summarize and interpret some of their results. I would also like to thank all the members of my family who put up my writing and sometimes even contributed to its formulation. I also thank everyone else who contributed to my writing. In addition to a number of unnamed, they are:
• Professor Jiri Bicak persistently guided my thoughts.
• Professor Michal Krizek gave me valuable information and assessment.
• MSc Jan Kaspar and Jan Slama taught me to express myself popularly.
And finally, I would like to thank you, dear reader, for your attention you gave me by reading this first part. Thank you.
20210330 >
